Ratio

Simplifying Ratios

To simplify a ratio, divide each part of the ratio by the same number each time, until they can no longer be divided.

Example: Write the ratio $\textcolor{red}{18}:\textcolor{blue}{60}:\textcolor{limegreen}{24}$ in its simplest form.

We can divide each number by $3$ and then by $2$ (or just by $6$):

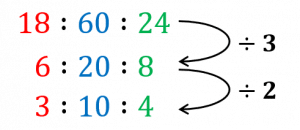
This cannot be simplified anymore to make whole numbers, so the ratio is in its simplest form, which is $\textcolor{red}{3}:\textcolor{blue}{10}:\textcolor{limegreen}{4}$

Solving Questions Involving Ratios

There are various types of questions involving ratios that you will encounter.

Example 1:  Olive makes her tea by adding $\textcolor{blue}{1}$ part milk to $\textcolor{red}{7}$ parts hot water $(\textcolor{blue}{1} : \textcolor{red}{7})$. If Olive uses $30$ ml of milk, how much hot water does she use?

The amount of milk used is $\text{\textcolor{blue}{1 part}} = \textcolor{blue}{30 \text{ ml}}$

Olive uses $7$ parts of hot water to every part of milk.

So,

The amount of hot water used is $\textcolor{red}{\text{7 parts}} = \textcolor{red}{7} \times \textcolor{blue}{30} = \textcolor{black}{210 \text{ ml}}$

Example 2:  Simon and Luca sell some items at an auction. They make $£450$ in total.

They split the money they make respectively in the ratio $\textcolor{red}{4}:\textcolor{limegreen}{5}$. Work out how much money each of them receive.

The ratio tells us that there are

$\textcolor{red}{4} + \textcolor{limegreen}{5} = 9$ parts

$\textcolor{red}{4}$ of these go to Simon and $\textcolor{limegreen}{5}$ of these go to Luca.

So, find how much $\textcolor{purple}{1}$ part of $£450$ is worth, by dividing the total number of parts, $9$

$\textcolor{purple}{\text{1 part}} = £450 \div 9 = \textcolor{purple}{£50}$

So,

$\text{Simon's share} = \textcolor{red}{4 \text{ parts}} = \textcolor{red}{4} \times \textcolor{purple}{£50} = \textcolor{black}{£200}$

$\text{Luca's share} = \textcolor{limegreen}{5 \text{ parts}} = \textcolor{limegreen}{5} \times \textcolor{purple}{£50} = \textcolor{black}{£250}$

Calculating Total Amounts

You can use ratios to calculate total amounts, using the following steps:

Step 1: Calculate the value of one part (you may be given this in the question).

Step 2: Calculate the total number of parts.

Step 3: Calculate the total amount, by multiplying the value of one part by the total number of parts.

Example: A basic dough is made by mixing $\textcolor{blue}{3}$ parts of Greek yoghurt and $\textcolor{limegreen}{4}$ parts self-raising flour.

$160$ g of self-raising flour is used. How much dough is made in total?

$4$ parts self-raising flour is $160$ g, so

$\textcolor{purple}{\text{1 part}} = 160 \div 4 = \textcolor{purple}{40 \text{ g}}$

The total number of parts is

$\textcolor{blue}{3} + \textcolor{limegreen}{4} = \textcolor{red}{7}$

So, the total amount of dough made is

$\textcolor{red}{7} \times \textcolor{purple}{40 \text{ g}} = \textcolor{black}{280 \text{ g}}$