Compound Growth

Interest

Interest is a percentage of some money that is then added on to the total amount of money. For example, when you put money in a savings account it generates interest.

Example: Youri puts \textcolor{orange}{£4800} into a savings account that pays \textcolor{blue}{5\%} interest per year. How much money does Youri have in his savings account after 1 year?

Calculate \textcolor{blue}{5\%} of \textcolor{orange}{£4800}: 0.05\times £4800 = £4800 \div 20 = £240

Add this on to £4800: £4800+£240=£5040

This could be done in one step: 1.05\times £4800=£5040

Compound Interest

Compound interest is interest on money, which includes previous interest that has already been applied. For example, money saved in a bank account may earn interest every year (‘per annum’), so the following year’s interest will be calculated by taking into account the interest that was earned in the previous year.

Example:  Tara takes out a loan of \textcolor{orange}{£700} from a loan company, who charge \textcolor{blue}{15\%} compound interest every month. Assuming Tara doesn’t pay back any of the loan, how much will she owe after 2 months?

After 1 month: 1.15\times £700=£805

After 2 months: 1.15 \times £805=£925.75

Money in the Real World 

Many questions require you to work with money in real world contexts. For example, you may be asked to evaluate a person’s budget, which may come in the form of a table or a list. Furthermore, you may also be asked to calculate the amount of tax someone needs to pay or how much they have left after income tax.

Example: Lauren has just moved into a new house and his calculating how much money she can put into a savings account each month.

Her monthly income after tax is \textcolor{red}{£1700}. Using the table below, calculate how much she can put into a savings account each month.

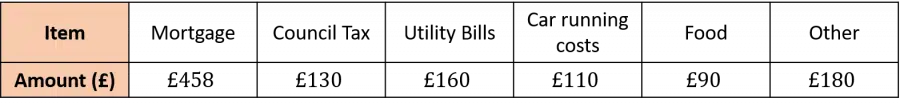
Adding up all her monthly expenditures: £458+£130+£160+£110+£90+£180=\textcolor{orange}{£1128}

Subtracting this from her monthly income: £1700-£1128=\textcolor{orange}{£572}

So she can put \textcolor{orange}{£572} into her savings account each month.

Example: Jamie earns \textcolor{red}{£27500} a year before tax. He isn’t taxed taxed on the first \textcolor{blue}{£12500} he earns (personal allowance), and then pays \textcolor{limegreen}{20\%} tax on all income over £12500. How much will Jamie earn after tax in one year?

Taxable income: £27500-£12500=\textcolor{orange}{£15000}

Tax on taxable income: 0.2 \times £15000=\textcolor{orange}{£3000}

Amount earned after tax: £27500-£3000=\textcolor{orange}{£24500}